The following table shows the ages of the patients admitted in a hospital during a year:

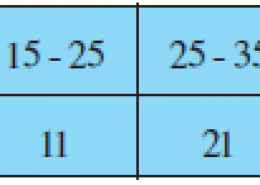
To find the class marks (xᵢ), the following relation is used.
xᵢ = (Upper Limit + Lower Limit)/2
Taking 30 as assumed mean (a), dᵢ and fᵢdᵢ are calculated as follows.

From the table, we obtain
∑fᵢ = 80, ∑fᵢdᵢ = 430 and a = 30
mean (X̄) = a + (∑fᵢdᵢ / ∑fᵢ) = 30 + (430/80) = 30 + 5.375 = 35.375 = 35.38
Mean of this data is 35.38. It represents that on an average, the age of a patient admitted to hospital was 35.38 years. It can be observed that the maximum class frequency is 23 belonging to class interval 35 -45.
Modal class = 35 – 45
Lower limit (l) of modal class 35
Frequency (f₁) of modal class = 23
Class size (h) = 10
Frequency (f₀) of class preceding the modal class = 21
Frequency (f₂) of class succeeding the modal class 14
Mode = l + ((f₁ – f₀)/(2f₁ – f₀ – f₂)) × h = 35 + ((23 – 21)/(2 × 23 – 21 – 14)) × 10 = 35 + 2/11 × 10 = 35 + 1.81 = 36.81
Mode is 36.8. It represents that the age of maximum number of patients admitted in hospital was 36.8 years.

Here is the video explanation of the above question😃